A rigid body is a solid object that does not distort when external forces act on it.  In other words, a rigid body keeps its shape.  This idealization is convenient for many types of approximate mechanical seal calculations.

Of course, in reality, mechanical seal components bend, twist, deform, etc. as can be readily shown by experiment or Finite Element Analysis (FEA).

In the field of mechanical seals, rigid body rotation does not mean the rotational motion of the seal components as mounted on a rotating shaft.  Instead, rigid body rotation (sometimes simply called “rotation”) is taken to mean the very slight angular displacement of the seal shape with respect to its normal position.  The “rotation” is considered to take place around the centroid of the shape and is calculated based on simple ring theory.

Figure 1 shows the distribution of pressure and concentrated force in a typical seal ring.   To determine the “rotation”, these forces are resolved into moments about the centroid of the shape.

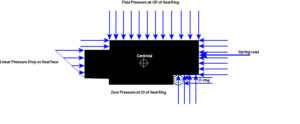
Figure 2 shows a “negative” rotation about the centroid.  The angle shown is greatly exaggerated; typically, the angle is only a few minutes of a degree.  Negative rotation results in OD contact and a diverging leakage path when the liquid is at the OD of the seal ring.  For many conventional pusher seal shapes, OD pressure tends to produce negative rotation.  The magnitude of the face angle is significantly influenced by the depth of the O-ring groove and the location of the face OD and ID with respect to the centroid.

Figure 3 shows a “positive” rotation about the centroid; again, the angle is greatly exaggerated.    Positive rotation results in ID contact and a converging leakage path when the liquid is at the OD of the seal ring.  For many conventional pusher seal shapes, ID pressure tends to produce positive rotation.  The magnitude of the face angle is significantly influenced by the depth of the O-ring groove and the location of the face OD and ID with respect to the centroid.  However, usually positive rotation is produced by thermal effects instead of pressure effects because the seal face is hotter than the seal body.

Figure 4 shows an exaggeration of a distorted seal ring.  This type of distortion must be calculated using FEA and not simple ring theory.

The simple rigid body rotation calculation can provide reasonable estimates for face angle, especially with regard to the effects of pressure.  The accuracy of the rigid body rotation calculation is reduced for long slender complex shapes and, in fact, can be misleading.  For example, it is possible for a shape to have a small negative face angle when considering only rigid body rotation but, in fact, distortion effects might result in a net positive face angle.